Here’s something enticing and strange: there are two “cut and paste” invariants of the same group which are equal to dual zeta values!

• the euler characteristic \( \chi(SL_2(\mathbb{Z})) = \zeta(-1), \) and
• the tamagawa measure of \( \mu(SL_2(\mathbb{Z})\backslash SL_2(\mathbb{R}) = \zeta(2)\).

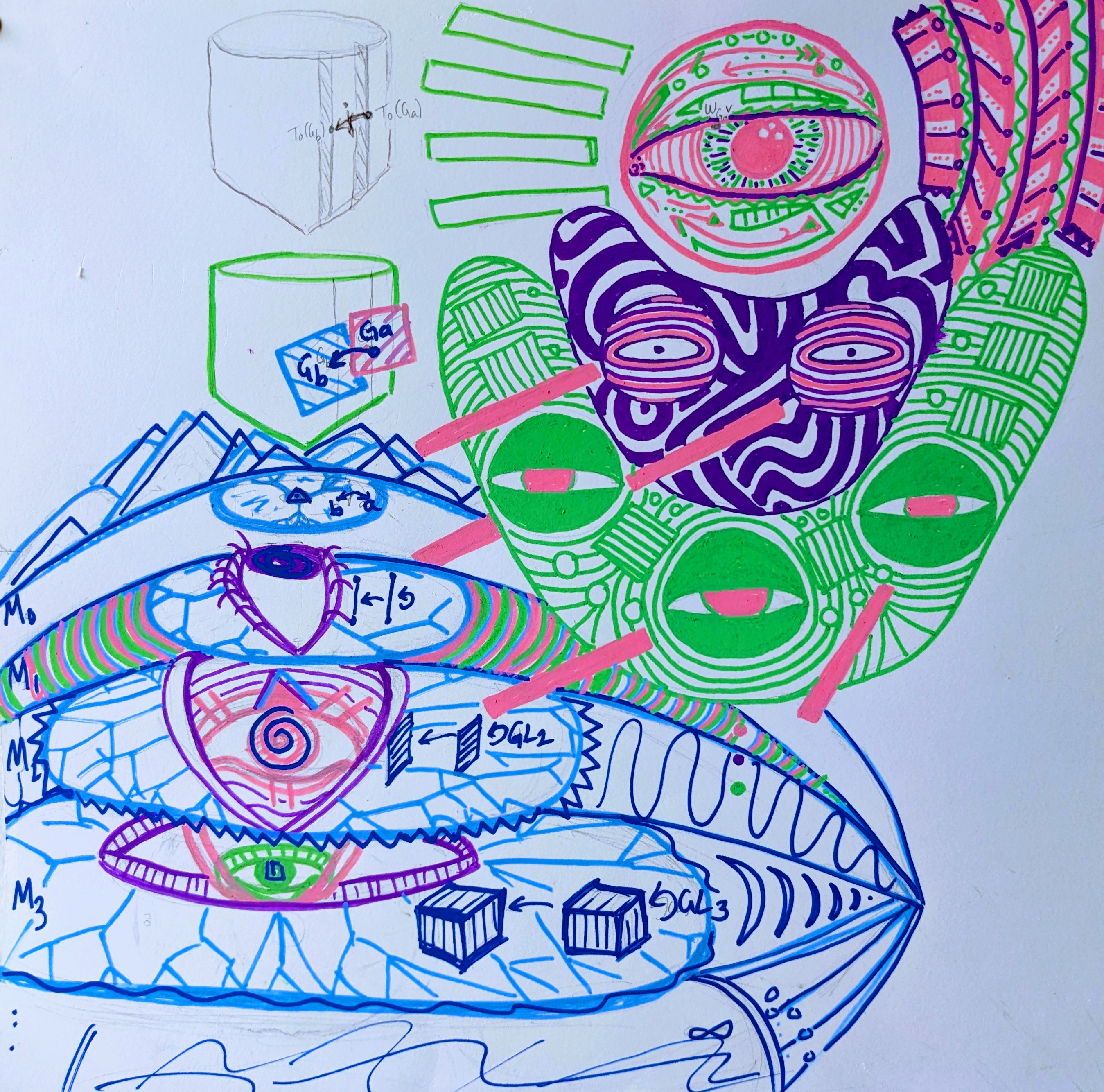This drawing is an old drawing I made when I was preparing for my qualifying exam in my second year of grad school at Northwestern. It is the crystalline period map. The tower to the left is the “Lubin-Tate” tower, the deeper it goes the more level structure. In the upper right corner there is projective space, and the “cat like” creatures below are moduli stacks of curves. I always draw the moduli stack of elliptic curves as a cat because it has 2 stacky points (its ears) and a third stacky point if you compactify “the sock” of the fundamental domain (its tail). At the time I was working on what became my PhD thesis: using moduli stacks of curves with marked points to understand moduli stacks of formal groups with level structure.

Cech Covers (click the link to listen to us). I wrote this song with my beloved old room mate Christian Gorski in my last year of grad school while I was wrapping up my thesis. For weeks, I was doing nothing but computing etale sheaf cohomologies of ramified covers of the projective plane. I would decompose sheaves on these curves by cutting around the neighborhood of ramification point (the stomach), and capturing the properties of the ramification group from the ramification point (the heart). The gluing back datum was the punctured neighborhood of the sheaf.

I have been working recently to counter the writers block that has formed insidiously from an unhealthy creeping perfectionism. In order to do this, I will post some old art and music which at the time I felt was “not good enough to share” or “inappropriate for a professional mathematician to have associated to them.” But all of these labels and caring too much about what other people think – I am sick of them. I don’t want them in my value system. I just want to be my full authentic self. So I will be. Enjoy!

We outline connections between the gut microbiome, autoimmune conditions, neuropathic pain, eye pain, chemical intolerance, and a specific set of “overactive” mental illnesses. All seem to be connected to a sensory processing disorder. This is joint work with Luca Estinto.

This post is written together with Josh Mundinger. Last time, we compared the Harish-Chandra isomorphism \(Z(U\mathfrak g) \cong (\text{Sym} \mathfrak h)^{W,\cdot}\) for \(\mathfrak g= \mathfrak{sl}_2\) to the Duflo isomorphism \(Z(U\mathfrak g) \cong (\text{Sym } \mathfrak g)^{\mathfrak g} \cong (\text{Sym} \mathfrak h)^W\), and found that they differ exactly by a translation by \(\rho\). In this blog post, we study just the Harish-Chandra map \(Z(U\mathfrak g) \to \mathbb C[\mathfrak h]\), using the Fourier transform to explain why the image is invariant under the dot action \((W,\cdot)\). Recall that the Harish-Chandra map sends \(z \in Z(U\mathfrak g)\) to the action of \(z\) on the Verma module \(M_\lambda\). The dot action of \(W\) is defined by \(w \cdot \lambda = w(\lambda + \rho) - \rho\). Thus, for \(\mathfrak sl_2\), we need to show that the center of \(U\mathfrak sl_2\) acts by the same scalar on \(M_{\lambda}\)and \(M_{-\lambda - 2}\).

This post is written together with Josh Mundinger. We seek to understand the relations between \(1/2\)’s that appear across mathematics. From the Riemann Hypothesis to the L2 norm, we aim to see the myriad and enticing ways this unfurls; each instance of \(1/2\) connected in an anarchic network of equals. In this blog post, we examine a specific example arising in representation theory: the center of the universal enveloping algebra \(U\mathfrak g\) of a Lie algebra \(\mathfrak g\).

Let’s compute the automorphism groups of some curves. Often presented as an insurmountable task, we must couragously go forward. There are many ways to do this! We will be using 3 different algorithms, so choose according to your taste. If you do not have SageMath installed on your computer, you can use the sagecell emulator.

Please enjoy my webcomic on what it feels like to run into a concept for the first time. I will be updating panel by panel, adding each one to this post as I make them. Here begins the adventures of Massey into the unknown.

I thought it might be of interest to homotopy theorists to learn how the Lubin-Tate action is relevant in the Hecke orbit conjecture. Here is a beginner friendly summary. heckeorbitshomotopy

This paper of Honda was very hard for me to track down. I found it in a retired library volume in a thrift store in England. It was not previously available digitally. Hopefully, this digital copy will make it easier for others to enjoy Honda’s incredible insight and understanding of how to create power series in one variable with high amounts of arithmetic information, and in general of higher height formal group laws.

Here are the texed notes for my 1 hour oberwolfach talk (the key thing I left out is Cartier duality). I included Manin’s original approach, and remark on some details I find left out of most sources.

Here is an incomplete proof that varieties are fiber bundles of formal disks over their deRham Stacks. The fact makes intuitive sense, the deRham stack is the variety without infinitesimal data, and then by adding the infinitesimal data (formal disks) back in, you recover the result. However, the fact that you can build anything non infinitesimal out of formal disks fills me with confusion and awe.

Here, \(A\) is any abelian variety. This post consists of the backstory of my latest paper with Dami Lee and something interesting I learned about the relationship between the size of \(Aut(A)\), and the number of principal polarizations \(A\) has.

This quarter (Spring 2018) we held a seminar on the Lurie-Gaitsgory proof of the function field case of the Tamagawa conjecture (Jora Belousov, Grigory Kondyrev, Yifeng Liu, and myself). I also started learning the uke, and I wrote a song for each talk (many use the tune of an existing song). I think the quality improved as I went on, so feel free to start from Lecture 8.

Texed notes of my reading course last quarter advised by Yifeng Liu, with fellow participants Grisha Kondyrev and Jora Belousov. Our goal this reading course was understand some of Scholze’s recent work with perfectoid space techniques, in particular the proof of the monodromy weight conjecture.

This is toward my understanding of the phrase “Why is height so important as an invariant? Because the height of a formal group law comes from the symmetry of the underlying variety.” In short — high amount of symmetry in the underlying abelian variety implies a high height of its formal group law (the converse is NOT true, if this was true, Elkies’s supersingularity theorem would be false).

Motivational Sidenote: This is part of my project in attempting to understand the notion of height (in formal group law theory) in terms of the symmetry of the underlying variety.

These notes began as my way of attempting to understand examples of abelian varieties (over finite fields) whose formal group laws posess higher height. Indeed, the notion that the height of a formal group law encodes the symmetry of the underlying abelian variety is a notion that I found irresistibly attractive to attempt to make precise and explore with examples.

I wrote my Master’s thesis. This thesis is meant to fill a gap in the literature, for those starting out on their first larger calculations (ones which are too large for normal paper), and to emphasize some crucial differential finding tricks often left unpublished, or abandoned shivering in a footnote or side comment. This exposition will be repetitive, there will often be multiple proofs of the same things – the aim is to teach methods used by active topologists by showing them in action.

Beauty wilts and love will flucture
what remains is hidden structure
blushing primes sly chiding knots
intertwine betwixt our thoughts

\(“(B-1)^n = B^n”\)

Thanks to Mia Hughes and John Huerta for the helpful discussions on this topic.

What does an integer have to be?

Hello. I don’t want much, just looking for a nice Variety to spend my days with. If you apply, I’d like you to have a well understood group law that comes from some 3-fold symmetry, but I’m a simple girl, easy to please, and I don’t need your group law to be all fancy and closed — a group chunk (group law which closed at least locally to the origin) is fine by me. I’ll have to put you through an interview process to see if you’re group chunk gives me a formal group law which is height 3, but don’t worry, it’ll be painless. Please let me know if you have a friend that matches this description!

I was learning about linearized stability and was confused by where the magical linearized version of the equation was coming from. I finally understand it, and so stupidly simple so I want to tell you about it. First, I’ll motivate the question. If you don’t care about the motivation, just scroll ahead a bit.

A quick post before bed, an impressionist stroke on some nice things lurking in linear algebra. I love polynomials. They are the ultimate tools that make me feel like I’m touching something, calculating at the level of a polynomial is a good clean feeling. I want to show you that it is nice to think of a vector space over \(F\) as a \(F[x]\)-module (thanks Emmy Noether). Thanks to Semon Rezchikov for helping me get over a few bumps in grasping some of the following.

I posted earlier a query toward exploring the analogy between

Apollonius of Perga (262 BC) wrote an exhaustive treatise exploring conics. He presented a classification of conic sections by angle. I’ll show you a summary of what he did, and then a conceptually more pleasing and suggestive way to think about it.

This post assumes that you are familiar with the definition of Lie group/algebra, and that you are comfortable with the Lazard ring. Note: This is less of an expository post and more of an unfinished question.

I didn’t want to use a brute force method, so I thought for a while about how to compute the cup product of the Klein bottle. This is what I came up with. I thought I’d share it.

I recently encountered a result which seems to be analogous to the following result of Dirichlet, which I wrote in a few common forms to be more suggestive.

Today, I want to discuss the opposite procedures of deformations and contractions of complex-orientable cohomology theories.

I came across a free book giveaway with everything from Maugham’s tropical works to various Russian math titles which I couldn’t read.

Today, we’ll be talking about a relation between the discrete and continuous.

This post assumes knowledge of the definition of the oriented cobordism ring, as well as the equivalence (\piMU \simeq MU^(pt) =: MU^*), and familiarity with the Landweber exact-functor theorem._

I read Bill Thurston’s On Proof and Progress this morning. This led me to consider a few things I’ve learned this summer about the sociology and psychology of being a part of the mathematical community, which I figured I’d share on the off chance that you might find it encouraging or helpful.

My dear friend Alex Mennen and I had some fun this morning defining the orientation of a ring.

When you construct a particular sheaf over an elliptic curve and then continuously vary the elliptic curve, what happens to the sheaf? I’m not sure, so I am first trying to understand what group contractions mean for elliptic curves.

Thanks to Laurens Gunnarsen for his superb pedagogy and for this amazing explanation on the incredible depth of connections springing from the Sperner lemma. All errors are mine not his. This started with a chain of events, sitting in on number theory seminars and encountering Abel’s differentials of the first and second kind, interest in the dessin, and led up to asking Laurens:

This question had been bugging me for a while, and I have been unable to find a source that is suited to the beginning topologist. Eric Peterson kindly answered this for me, and I found his explanation so astoundingly beautiful that I wish to share on the off chance that you, dear reader, will similarly appreciate this visually rich narrative. All errors are mine and not his.

Before I get into this post, allow me to give a bit of back story.

Euler was a swashbuckler. He considered the following series, nevermind that it sums to infinity, let’s see what we can do with it!

Usually, besides the information preserved by the formal group law of the elliptic curve, we can’t see any information about the elliptic curve when looking at the output of its associated cohomology theory. The formal group law only* remembers if the curve was singular/supersingular, and the characteristic of its field.

Inspired by the extraordinary expository style of Dr. Kazuya Kato, I’ve started reading parts of a (translated) Japanese children’s book when I’m stuck on a tough paper or concept — revisiting the concept with such a dreamlike world in mind usually unfolds an illustrative perspective. A misty world which begs to be put into firm ground via prolonged formal and concrete afterthought.

Spectra come from the need for negative dimensional spheres — the need for a category where suspension has an inverse and not just an adjoint!

This post assumes familiarity with the Landweber exact functor theorem, elliptic genera, and bordism theories.

Eine deutsche Übersetzung des folgenden Abschnitts befindet sich hier.

_This post assumes that you’re familiar with the definition of a prime ideal, a local ring, \( R_{(p)} \), the sphere spectrum, \(\mathbb{S}\), and the Lazard ring, \(L\). During a talk Jacob Lurie gave at Harvard this April, he labeled the moduli space of (1-d commutative) formal group laws as \(\text{Spec }\mathbb{S}\).

Here’s a flowchart I made while preparing for an upcoming talk. I fear that it may be hard to follow without being already familiar with the story, but there’s little harm in posting it. Maybe it’ll help someone navigate the literature.

This post assumes familiarity with formal group laws, the definition of exact sequences, the motivation of the Landweber-Ravenel-Stong construction, that the exactness axioms are one of the generalized Eilenberg-Steenrod axioms, and the fact that formal group laws over \(R\) are represented by maps from the Lazard ring to \(R\).

Ich lerne Deutsch. Bitte, vergizieh mir und meine Unwissenheit der deutschen Grammatik. In der Studie von Gruppen mit topogischen Struktur, wir haben die globale Objekt (die Gruppe) mit eine lokalen Objekt (die infinitesimale Gruppe) ersetzen. Wir betrachten dieses Spiel folgt vor:

There are likely inaccuracies in this post, as I am just beginning to learn the basics of algebraic geometry. Constructive criticism is strongly encouraged.

There are likely inaccuracies in this post, as I wrote it quickly and am just beginning to learn the basics of algebraic geometry. Constructive criticism is strongly encouraged.

I’ve resurrected this post from my draft graveyard after chatting with Chas Leichner about the lightly related notion of domain theory, and the interaction between computation and topos theory.

I’ll assume that you know what a line bundle is and are comfortable with the following equivalences; if you aren’t familiar with the notation in these equivalences, John Baez might help. Note that integral cohomology := cohomology with coefficients in (\mathbb{Z}).

Edit: When I say cobordism, I mean oriented cobordism unless stated otherwise. Also note that I accidentally flip-flopped \(\Omega^*\) and \(\Omega_*\) — \(\Omega^n\) should be cobordism classes of maps from manifolds of codimension \(n\) to \(X\), and \(\Omega_n\) is cobordism classes of maps from manifolds of dimension \(n\) to \(X\).

We have a functor Spec from Ring to Schemes:

In singular cohomology, the first chern class of two tensored line bundles (c_1( A \otimes B) = c_1(A) + c_1(B)) is the additive formal group law, (F(x,y) = x + y).

I noticed an informal “recipe” for taking a type of object and constructing invariants (of the object). It’s been useful for removing the feeling of “what, why? where did that come from?” when learning new constructions that fit this recipe. Hopefully it will help you!

When I define a polynomial, I am simply handing you an indexed collection of coefficients.

And I sit here, undeserving,
in a small flat
staring out the window, dear.
Thinking of patterns, and sometimes of regrets.
Staring at symbols, trying to see what the author saw.
Trying to be them, just for a second,
to glimpse the beauty they’d uncovered
by sitting in a small flat,
staring out of their window
thinking of patterns, and sometimes of regrets.

In Segal’s Bourbaki talk on Elliptic cohomology, he mentions offhandedly that:

This collection of notes resulted from my desire for an intuitive grasp of basic concepts in topos theory, and is meant to complement to a standard introduction to topoi (i.e., this is pedagogically unsound).

Disclaimer: This exposition is profane. It is the result of me trying to be productive during a raging headache, and ending up with comic relief.

A 10 year old girl and her father sat in the back of my car as I drove them home after Thanksgiving.

This post assumes knowledge of fiber bundles, the group action functor, groupoids, and basic vector calculus. I am in the process of learning the topics discussed below, and I deeply appreciate constructive feedback.

There are at least three distinct conceptual roles which vectors and vector spaces play in mathematics:

This quick post assumes basic knowledge of Lie algebras and category equivalence. I am new to category theory, and appreciative of constructive feedback.

Treating spaces as fiber bundles allows us to tame twisted beasts. Most of spin geometry is phrased in the language of fiber bundles, and this post will begin to introduce that language — extremely powerful in its simplicity.

Group(oid) theory is the study of symmetry. When we are dealing with objects that appear symmetric, group theory assists with analysis of these objects. The label of “symmetric” is applied to anything which stays invariant under some transformations.

Mathematics is a huge subject.

Thanks to Dr. James Hughes, Matthew Lynn, Chris Walker, Chas Leichner, Alex Ray, Alex Zhu, Dr. Cornelia Van Cott, Paul Sebexen, Colin Aitken, Chuck Moulton, Sebastien Zany, and Nick for joining me in playing with this problem.

My topology curriculum is an example of How To: Learn a New Discipline.

Already, criticism has be thrown at me for deciding to focus on pure mathematics. I am often told (with the best intentions) that I should go back to doing applied math/engineering. I’m guessing that other people who’ve decided to study theoretical subjects suffer from a similar lack of respect.

My mentor, Eric Weinstein, made up a lovely analogy which he permitted me to share.

Topological Maps in Robotic Navigation

The context of this post is my pressure ulcer prevention research.

There are many methods for manipulating living cells and pieces of dead ones. The method of choice is entirely context dependent.

Executive Summary

Adam and I built a sign for my desk.

In 2012, I researched computational materials science/physics for Dr. Dimitrios Papaconstantopoulos. The following is my semester report. All source code is listed at the end of the report.

My default mode of research consists of many projects at once, spanning multiple disciplines.

Diving Into Neuroprosthetics

I used to overcomplicate my todo system.

This post is an experiment in explaining math concepts via colorful hand-drawn diagrams.

I’m experimenting with committing past projects to github.

A pressure ulcer is an ulcerated area of skin caused by irritation and continuous pressure on part of the body. It starts as an area of skin damage which spreads to the tissues underlying the skin. In severe cases (Stage IV), there can be permanent damage to muscle or bone underneath the skin. Pressure ulcers are most common over bony prominences. Leading contributors to pressure ulcers: pressure, moisture, friction/shear and nutrition. Tissue is stressed between the bony mass and a rigid surface (such as a chair seat or bed mattress).

I recently switched my music vendor from Google Music to Spotify. To avoid manually searching for each song, I semi-automized the transition as follows.

tl;dr In order to build your knowledge base, start with a question you want answered and work back from it.

It is often the affliction of creative people that we have too many ideas and think of time as our most precious resource.

A lesson that every scientist (or any person in a fast-paced creative field) learns: be glad when we find out that our research idea has already been done.

I’ve just recently taken on a contract project. Adding that project onto my wheelchair and protein detector projects is a fun exercise in multi-threading that contributed to a recent realization.

I’m regularly asked about the setup of my laptop: a System76 Gazelle Professional named Felix running Ubuntu 14.04. Writing a return to that query produced the following:

I tried a new type of gradient shading and ended up with a mix of photorealism and comic book style.

This is a summary of a talk I attended at Stanford by Douglas Hofstadter (well known for his authorship of Gödel, Escher, Bach: An Eternal Golden Braid).

I was recently asked an interesting question in an interview:

This is a paper I wrote my 3rd year of university as I was working on my Machine Learning-based Braille translator research. Enjoy!

I enjoy songwriting and have recently gotten into playing Third-Wave ska on my trombone. The basic premise of ska is to play an incredibly upbeat tune with shocking/macabre lyrics.

During an update to the server in September and an issue with the backup system, the site was corrupted. Some posts have been totally lost. All previous comments have been totally lost.

Name: Frodo Baggins

Here: have a script to generate plaintext hexagonal lattices for you when you’re feeling blue.

While going through past notebooks, I came across a table I’d compiled which covered basic enumerative combinatorics.

DISCLAIMER: This is the solution to Project Euler’s problem 15. Please attempt to solve the problem yourself before reading my solution.

Having a reliable method to control your fear and achieve focus quickly is indispensable. During one sleepless night reading Frank Herbert, I discovered my solution.

A surprising amount of Linux users are unfamiliar with how to force quit programs via the command-line. It is often that your only option to escape a process gone awry is using the term window.

Large scale doodling with tape is an interesting experience.

I wanted wall art for my office (\rightarrow) I made myself wall art with painter’s tape during a 30min break from working on my simulation. The triangle is fairly large, (h \approx) 130 cm (\approx) 4’4”

Katriona Guthrie-Honea, Austin Russell, and I recently brainstormed to come up with an introductory science kit. The goal of the kit is to create wonder and light the flame of curiosity; revealing that science is a series of fun puzzles and that magic is more beautiful when you understand how it is done.

Number theory is the cocaine of mathematics. The following resulted not from my intention to prove the conjecture, but from the drive to deeply understand its underlying structure.

Some of these may seem obvious; keeping their importance in mind is not so obvious.

I visited my friend, Megan Brusnahan, before I left for CA. She had a picture I’d drawn her in middle school (2009) mounted on her wall in a frame. I’d forgotten that I’d drawn this picture! It’s done entirely in black sharpie.

This quick post assumes a basic knowledge of categories and functors, which can be gained from a previous video post.

Get Weather on the Unity top panel in Ubuntu! Worry not, this tutorial doesn’t assume any term aliases.

Bra-ket notation is concise and useful.

I attended HackMIT 2013 and had an absolute blast! Kartik Talwar, Spencer Hewett and I make a fantastic team.

The internet went down at my house, and I decided to play with vpython again!

There is a connection between engineering and music. For the sake of simplicity, I will shorten “math, physics, computer science, mechanical & electrical engineering” to “engineering.”

I mentioned that I set my system font to Braille in a previous post. This tutorial generalizes to any font you can find in a .ttf format!

In a rush, so I figured I’d record an informal cover and leave all of the mistakes in. Not sure why it came out so low res!

Today, let’s have some fun playing with perspective rendering in Python! My graphics package of choice is VPython:

Disclaimer: This informal post assumes you are familiar with basic photonics and applied chaos theory.

Konzepte der Quantenmechanik durch den dunklen Nebel der Unwissenheit aufgefressen. Lass mich den Nebel eines verbreiteten Missverständnisses aufheben. Lass mich deine gegenwärtige Meinung ändern.

Want to type in another language but you love your US keyboard layout?

Project Unbreakable is a project run by Grace Brown, Kaelyn Siversky, Christina Dunlop, and Kerri Pang. It was created to raise awareness of the common nature of sexual assault and serves to alter the general sociocultural perception of rape. Disproving the regular assumption that rape is an uncommon, unfortunate occurrence that happens only to those who deserve it. The project is a composed of a collection of art submitted by survivors. These submissions are photographs of a survivors holding posters decorated with quotes from their attackers. Submissions also include quotes from others in reaction to survivors seeking help (e.g., “You deserved it,” “I don’t believe you”). This project features male rape survivors and showcases the reality that men can get raped. There is no discrimination as to who can participate in Project Unbreakable (“anyone who has experienced any form of sexual abuse, whether physical or emotional”). However, they do not accept admissions from children.

What is the difference between (\frac{d}{dx}) and (\frac{\partial}{\partial x})?

Have no idea what’s going on? Have no fear: Introduction to Visual Grade 2 Braille

Below is a quick attempt to summarize and extrapolate from an article on Optical Routing And Sensing With Nanowire Assemblies in a simple manner, without assuming the reader is deeply familiar with the esoteric lexicon of photonics and optoelectronics. I can’t freely distribute the article which inspired this post; check with your local university to find a copy. More information on the relevant article is post script.

Today, I wanted to code an efficient letter to Morse code translator in Python and whipped this up. I’ve found that a familiarity with many of Python’s lesser-known built-in functions is quite useful in situations such as this!

I learned braille for 3 reasons. The first is my hobby of picking something random and learning it. The second is because I wanted to learn touch typing. The third is because I often fell asleep while reading and left the light on. This way, I can read myself to sleep without a light on!

It is tempting for some to take all of the glory.

Problem: I wanted to use a different medium than sketching, and I wanted to personalize my laptop. Decals are way too expensive, the material to make them is super cheap.

Sometimes, when juggling a particularly busy lifestyle, a stand-alone Todo list isn’t enough. I find it useful to supplement my Todo system with a weekly schedule in order to quickly allocate Todos to each day.

Early on in their endeavors, most mathematicians and physicists teach themselves to handwrite the letters of Greek alphabet as quickly as the letters of the English alphabet.

I was recently approached to write an essay to convince (high school) art students that math is freaking excellent. This was the result:

Disclaimer: The following is only my opinion. I am not directly affiliated with the Thiel Fellowship or Thiel Foundation, but I did have the opportunity this summer to interact with and work with many of the fellows and other people involved in the community.

The ultimate mental image for transmitting and receiving the Morse alphabet (click on the image for larger view).

Have no idea what’s going on? Have no fear: Introduction to Visual Grade 2 Braille

Today’s dose of code will be less of a program and more of an advanced vim tutorial.

Today, as I was writing a post on sorting in vim, I issued myself a challenge.

If you’ve run into me in the past 2 days, I’ve squeaked at you and scribbled on my notebook “lost my voice! How are you?” As of this post, I am still mute. However, I will write it in the past tense to create a false sense of encouragement that my voice will return soon.

I am often asked how to stop procrastinating or asked how I get so much done. While I think I don’t get enough done, I will share some of my methods of crushing procrastination with mindfulness.

Due to my accelerated graduation, I’m taking a lot of general education requirements this semester.

The code on my blog will range in quality from “I’m waiting in line and have 10 minutes to code” to “I’ve been working on this all day.”

Let’s say you want to decorate an all-black surface, without damaging it deeply. If engraving and sharpies aren’t within your acceptable option set, I suggest duct-tape and an exact-o knife. Begin covering your surface with a duct tape canvas. Next, sketch your desired design on some paper (I suggest graph paper) and secure each piece of paper with scotch tape.

During a late-night conversation with Austin Russell, he stated that we are living in a simulation. Together, we discussed the job description of a Universe Debugger, and created a fun way to project the world onto coding principles and concepts.

Unfortunately, the current CoffeeScript docs do not support installation on the latest Ubuntu distro. To get around this, we must manually install the dependencies. Don’t worry, I’ve done most of the work for you.

The purpose of this post is to link you to a post I wrote for Cloudera’s blog:  What I Learned During My Summer Internship at Cloudera

Let’s say we have a plain text file, erdos.txt, with the following contents:

I was recently hanging out with some friends, and one of them brought out an old math problem sheet. This problem sheet was briefly passed around and then put away again. One of the problems was a cute math puzzle. This problem was…

Grep is a magical beast which can be used to make your bash scripts excellent. This post will give you a taste of its utility. Let’s say I have a file, temp.txt which contains two lines:

Using LIKE in a query is an order of magnitude faster than using REGEXP. The downside is that LIKE doesn’t offer the control and generality that REGEXP does.

Let’s say I’m trying to insert some information into a mySQL table using JDBC.

I’ve recently decided that I should put some of my projects on GitHub.

I used to drown in a sea of post-it notes and repetitive todo lists, my thoughts spread across unorganized Dropbox files, repetitive Evernote entries and various iPhone apps (Checklist, Notes, GoogleTasks).

Before today, the most Perl I’d written/read was dinky one-liners.

GeoIP uses a large database to find information about a given IP address or website. I went through a couple different package installations before it integrated with my programs successfully. The fairly simple process I settled upon to set up the functional package, pygeoip (python API for GeoIP), is as follows:

I have been drawing mainly hands for a while, so I figured I’d use my free time to sketch something different.

At work the other day, I was reading about Hadoop’s 5 daemons. The information wasn’t quite clicking, so I drew a picture to cement the concepts into my mind. (I’ve checked that all information regarding Hadoop in this blogpost is publicly available.)

I bring my lunch to work to avoid food poisoning (I have food allergies, not paranoia). To be able to make these lunches for work when exhausted, I’d like a list of what to pack for a set number of days.

I recently became stuck trying to create an animation which consists of a smooth rotation of a viewpoint around the Lorenz attractor. Methods I use for changing viewpoints with respect to surface objects were creating jerky, lagging animations when applied to my line plot.

This video also sneakily teaches you the phonetic alphabet.

I’m a big fan of the Lorenz Attractor, which, when plotted, resembles the half open wings of a butterfly. This attractor was derived from a simplified model of convection in the earth’s atmosphere. One simple version of the Lorenz attractor is pictured below:

This is a continuation of Matlab: Lorentz Attractor, however, these methods can be applied to any line plot or collection of points.

Let’s say we want to create an aesthetically pleasing visualization of 2 coaxial cylinders.

Doctor Who depicts the adventures of a humanoid alien, the Doctor, who roams the universe on a sentient spaceship. This spaceship is referred to as the TARDIS which is an acronym for Time and Relative Dimension(s) in Space. As a Time Lord, the Doctor is able to regenerate his body when he is near death. Each of his incarnations has their own quirks but otherwise share the memories and basic personality of the previous incarnations. The Doctor often brings human companions to accompany him on his quests through parallel universes and different dimensions. On these adventures he saves civilizations and rights wrongs. The Doctor regularly gains new companions and loses old ones. The companions provide a surrogate with whom the audience can identify, and they serve to further the story by manufacturing peril for the Doctor to resolve.

In which I play a bit, debunk some misconceptions, teach you the slide positions and embouchure (i.e., mouth shape), and show you how to safely bike with a trombone.

A video of me drawing a hand as my interpretation of Tolkien’s Elven Welcome plays in the background. Enjoy!

Let’s say you want to make a simple simulation of a sphere spinning in Matlab. First, you set the pop-up window to have the title ‘Spheres,’ the window to have black background, and specify said window’s position and size.

To celebrate the removal of my braces (a few months ago), I drew on my tenor trombone’s bell.

I recently saw an add for a product I am excited for (although most blind people I know prefer Siri):

I recently watched a video by MinutePhysics where he seems to prove that infinity = -1.

In my data visualization class, we had an assignment to create a “rainbow” (create and display 128 vertical stripes of color in one image, in RGB sequence). Something like this:

The poster I’m using to present my research creating CAMEL is finally finished (full-size version: CAMEL_poster).

I’m brushing up on my Java lately using a wonderful code practice site called CodingBat. Below are a few of the solutions I’ve found to their practice problems.

For warmups, CodingBat provides solutions. Some of my solutions differ from the provided.

Do you have trouble remembering how to properly steer when driving in reverse?

I created a video for my Modern Physics class explaining the DC Josephson Effect in less than 5 minutes. You might enjoy it, I sure enjoyed making it!

Disclaimer: I’m a mediocre chess player, I do not consider myself a chess expert. These simple tips are for beginners looking to surprise intermediate players during games.

Often, when cubing, I’m asked: Can you solve it with your eyes closed?

On the topic of groovy math, here’s some smooth algebra.

We use algebraic chess notation to represent chess positions without posting a full chessboard. This allows players to converse about chess positions clearly without a board in front of us. Imagine the chess board as a 2D plot. Below is the table I made to explain this notation in my paper How Stockfish Works: An Evaluation of the Databases Behind the Top Open-Source Chess Engine. I will post more about this paper when I’ve finished editing it.

Perl’s string handling is a beautiful thing. I’ll talk about grep in a later post.

I enjoy songwriting. Here are the lyrics to a silly, fun song I wrote a couple of months ago.

I think math shortcuts and tricks are groovy. Here’s an arithmetic trick to find the fractional form of a repeating decimal.

I was writing a letter to my grandma. In the letter, I included a poem. My Uncle asked if I would send him more poems.

I used Braille as a test language, but this is a framework to automate the decoding of any partially understood (ancient) language by creating probabilistic dictionaries.

I have an aversion to hard-coding. Hard coding is when you write out a long, elaborate code that could also be written with a dynamic loop. This usually limits the ability to easily adjust your own code in case you want to change something later (or re-use it). “Hard coding” refers to “rigidly” writing out things instead of keeping them dynamic.

I love programming and know a few programming languages. Every programmer I’ve met has a favorite language, however, most of us can code in more than one language. As I was revising the ‘Language’ portion of my resume, I became confused.

I enjoy drawing. Below are a few of them.

I’m currently researching machine learning using Braille as my language platform. There is a Research Symposium for Mason’s College of Science at the end of April.

I am creating this blog in order to keep my family and friends updated on my adventures. I often neglect to update those close to me on my daily life and changing interests, because of my tendency to get caught up in my schoolwork and research. I expect this blog an eclectic patchwork of explanations, project updates, doodles, and code.

I’m currently reading Algorithms Unplugged, lent to me by my friend and professor, Dr. Marr.